All the students are required to study a variety of shapes and methods for determining area and perimeter. One thing to keep in mind about two-dimensional shapes is that they have no volume. Only three-dimensional bodies or forms can have their volume computed. Every student should be able to compute any shape’s area, volume, and perimeter. These are the fundamental calculations that should be able to be made for certain shapes. Many different shapes can be found all around us. In this article, we are going to discuss in detail two of the most important three-dimensional shapes and they are rectangular prisms and triangular prisms.
Rectangular prism

source: made-in-china.com
The number of corners, edges, and faces is the first thing we consider in any shape. A rectangular prism is a three-dimensional object with eight corners, six faces, and twelve sides. It’s important to note that volume can only be calculated for three-dimensional shapes. As a result, a rectangular prism has three dimensions. In a rectangular prism, every two faces that are opposite each other are congruent. The fact that the base and top of this design are always rectangles is vital to remember. As, because of the rectangular base only, its name is given as a rectangular prism.
The volume of a three-dimensional body is the most significant item to compute. A rectangular prism’s volume is simple to calculate. The area of the base, which can be determined using the formula for the area of a rectangle, is required to compute the volume of this shape. Once the area of the base has been determined, the volume of the rectangular prism can be calculated by multiplying the height of the prism by the area discovered. If the prism is rectangular, the base is denoted by b, the length by l, and the height by h. The volume is then calculated by multiplying all three quantities using the procedure described above. That is, l*w*h determines the required volume. Every child should remember this formula to easily solve problems related to the volume of a rectangular prism.
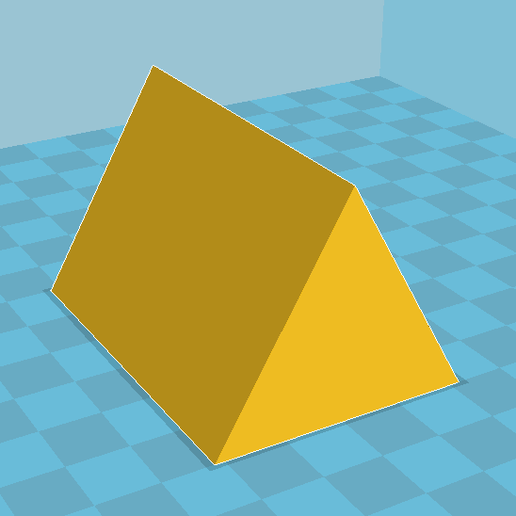
Triangular prism
source: pinterest.com
There are two triangular bases and three rectangular faces in this form of a prism. In a triangular prism, the total number of corners is six, the total number of edges is nine, and there are a total of five faces. The space occupied by a triangular prism is precisely proportional to its volume. This shape’s volume is easy to come by. A triangular prism’s volume can be calculated by multiplying the area of its triangle-shaped base with the height of the prism. The type of base triangle is an important consideration to make when determining the volume. The base triangle’s area is computed using the formula for that particular triangle. If the base is an equilateral triangle, the equilateral triangle’s area formula should be applied. In the same way, if the base is of a different kind, the formula for that triangle should be applied and then multiplied by the prism’s height to get the volume. It is the simplest way of calculating the volume of this kind of prism.
Every student should be able to calculate and understand the volume of a rectangular and triangular prism. In the preceding essay, we attempted to adequately explain both the shapes and the volume calculations to pupils. These notions are not only useful in our academics, but also in our everyday lives. As a result, pupils must fully comprehend them. Cuemath can assist anyone who is having difficulty learning and comprehending mathematical topics. Cuemath has already made a significant impact in the field of mathematics education.